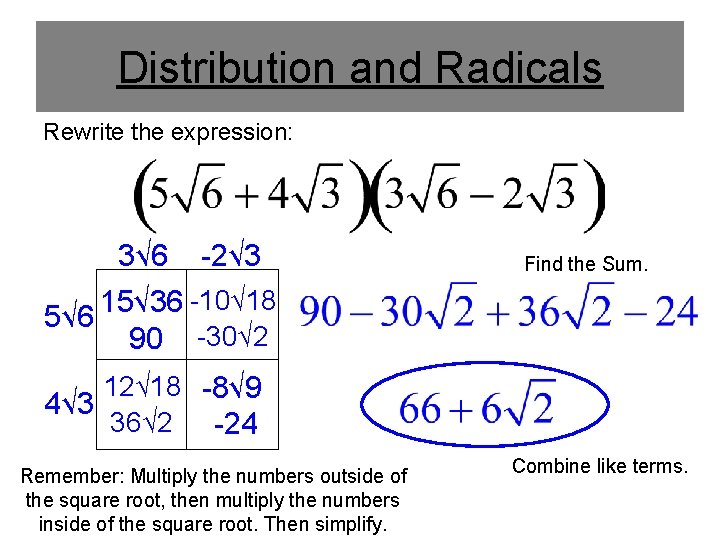
上 simplify 2√3/3-√3/6 687137-Simplify 2 root 3/3-root 3/6

Algebra Simplify Calculator Step 1 Enter the expression you want to simplify into the editor The simplification calculator allows you to take a simple or complex expression and simplify and reduce the expression to it's simplest form The calculator works for both numbers and expressions containing variables Step 2Example Sides of a right triangle are cm and 21 cm, find its hypotenuse Pythagoras Formula a 2 b 2 = c 2 c is the length of the hypotenuse a, b are the lengths of the other two sides (you can assume any length as a or b ) Let AB = a = , BC = b = 21 Putting values in the formula 2 21 2 = c 2 400 441 = c 2Click here👆to get an answer to your question ️ Simplify 2√(6)√(2)√(3) 6√(2)√(6)√(3) 8√(3)√(6)√(2) Solve Study Textbooks Guides Join / Login

Simplify Each Of The Following Expressions 3 3 2 2 3 3 3 3 5 2 5 2 5 2 Youtube

Simplify 2 root 3/3-root 3/6

Simplify 2 root 3/3-root 3/6-2 3 ( 2 − 6) Use the distributive property a ( b c) = a b a c 2 3 ⋅ 2 2 3 ⋅ ( − 6) = 4 3 − 2 3 6 For 2 3 6, factor 6 as 3 ⋅ 2 and applying the radical rule a b n = a n b n, 3 ⋅ 2 = 3 2 By applying the exponent rule, 3 ⋅ 3 = 3 1 2 ⋅ 3 1 2 = 3 2 2 = 3 So we have 2 2 ⋅ 3 = 6 22 3 ⋅ 2 2 3 ⋅ ( − 6) = 4 3 − 2 3 6 For 2 3 6, factor 6 as 3 ⋅ 2 and applying the radical rule a b n = a n b n, 3 ⋅ 2 = 3 2 By applying the exponent rule, 3 ⋅ 3 = 3 1 2 ⋅ 3 1 2 = 3 2 2 = 3 So we have 2 2 ⋅ 3 = 6 2 And going back to the original equation, we have 4 3 − 6 2 or 2 ( 2 3 − 3 2) Revathi

Real Numbers And Radicals

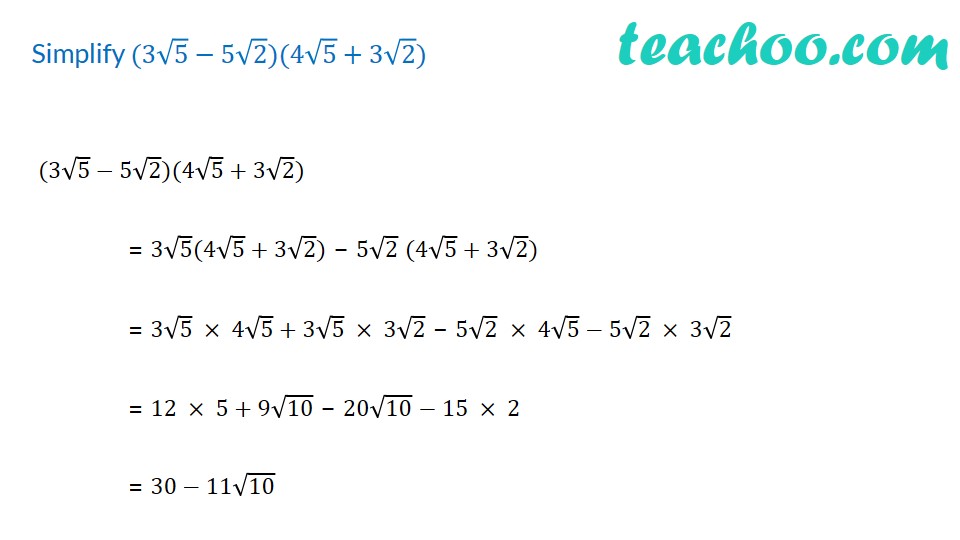
 Answer 5√37√22 Stepbystep explanation 3√2√6−√32√3√6 24√3√6−√2 Bringing the like terms 3√2√62√3√6−√2−√32 Now we apply the rule which says √a x√b = √ab 3√ (2x6)2√ (3×6)√2√32In this math video lesson on Adding and Subtracting Radical Expressions, I simplify 2√33√27 #simplify #radicalexpression #minutemathEvery Month we have aSolution Given, the expression is 3√3 2√27 7/√3 We have to simplify the expression 2√27 = 2√9 × 3 = 2 (3√3) = 6√3 Rationalising the denominator, 7/√3 = (7/√3) × (√3/√3) = 7√3/3

T hen you can simplify √ 25 to 5 Use any root explorer to edit /system/build 1) 96 4 6 2) 216 6 6 3) 98 7 2 4) 18 3 2 5) 72 6 2 6) 144 12 7) 45 3 5 8) 175 5 7 9) 343 7 7 10) 12 2 3 11) 10 96 40 6 12) 9 245 63 5 13) 7 600 70 6 14) 5 45 15 5 15) 5 180 30 5 16) 3 405 27 5 17) 2 36 12 18) 9 125 45 5 19) 8 27 24 3 ) 12 1764 504 21) 3 900 90 22Luck77 Luck77 3 weeks ago Mathematics College answered Simplify fully 2√3 x 3√8 1 See answer Advertisement Advertisement Luck77 is waiting for your help Add your answer and earn points heysdaddias123 heysdaddias123 Answer 12√6Free PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystep

NCERT Solutions For Class 12 Maths;Ayushmanyadav ayushmanyadav 1 minute ago Math Secondary School 6 Simplify (3√3)(2√2)² ayushmanyadav is waiting for your help Add your answer and earn points New questions inSimplify (2/3)^3 ( 2 3)−3 ( 2 3) 3 Change the sign of the exponent by rewriting the base as its reciprocal (3 2)3 ( 3 2) 3 Apply the product rule to 3 2 3 2 33 23 3 3 2 3 Raise 3 3 to the power of 3 3 27 23 27 2 3 Raise 2 2 to the power of 3 3

What Is The Relation Between Following Numbers 3 6 27 5 8 39 1 7 48 3 4 Quora

How To Simplify 16 5 2 7 Of 2 6 3 2 1 3 Quora

 Ex 15, 2 (i) Chapter 1 Class 9 Number Systems (Term 1) Last updated at by Teachoo This video is only available for Teachoo black usersClick here👆to get an answer to your question ️ Simplify (3 √(3)) (3 √(3)) You can simplify the fractional exponent just like any fraction 3 6 = 1 2 ∴ 21 2 This can also be expressed as √2 We can prove this by the following We know that the square root of a number, when multiplied by itself equals the number So

Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2

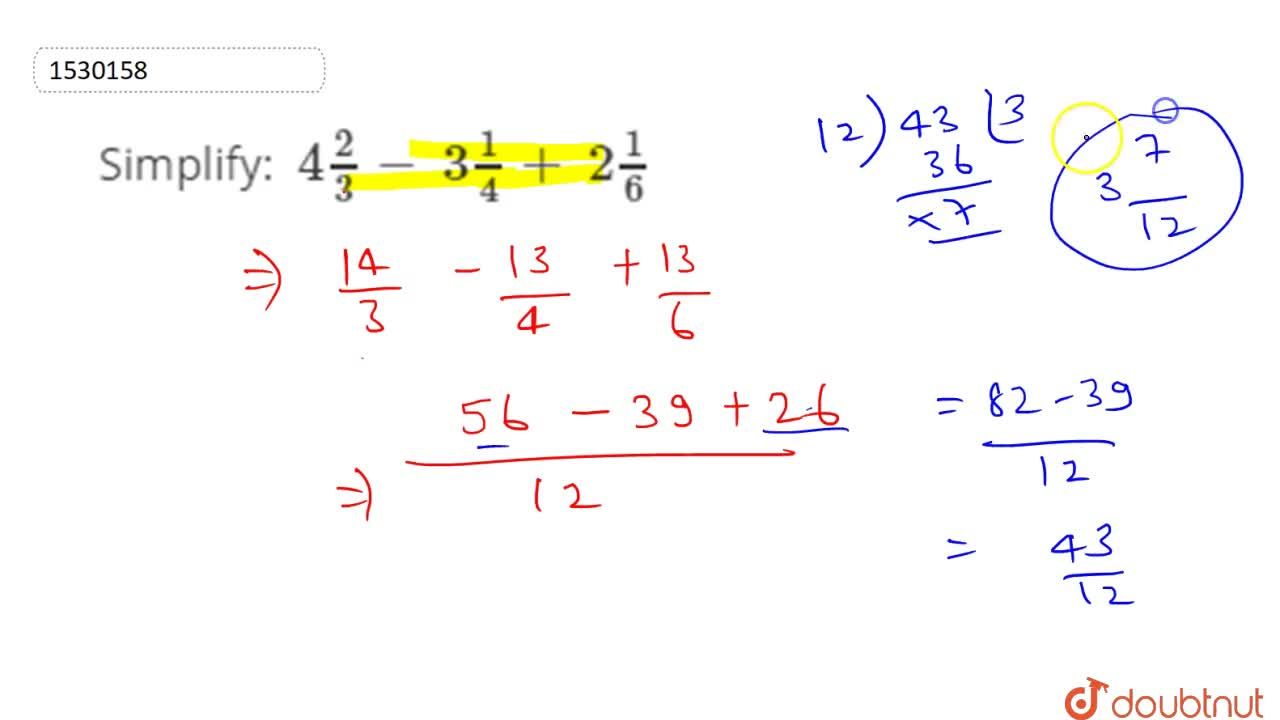
Simplify 4 2 3 3 1 4 2 1 6

 height, in feet Write a quadratic regression equation for this set of data, rounding all coefficients to the nearest hundredth Using this equation, find the height, to the nearest foot, at a time of 51 seconds Time in Seconds (x) Height in Feet (y) 0404 6464 0808 1212 1616 2323One Time Payment $1299 USD for 2 months Weekly Subscription $249 USD per week until cancelled Monthly Subscription $699 USD per month until cancelled Annual Subscription $2999 USD per year until cancelledNCERT Solutions Class 9 Maths Chapter 1 Exercise 15 Question 2 Summary Thus, the simplified values of (3 √3) (2 √2), (3 √3) (3 √3), (√5 √2)², and (√5 √2) (√5 √2) are 6 3√2 2√3 √6, 6, 7 2√10 and 3 respectively

Topic 4 Real Numbers Rational Numbers To Express A Fraction As A Decimal Divide The Numerator By The Denominator Ppt Download

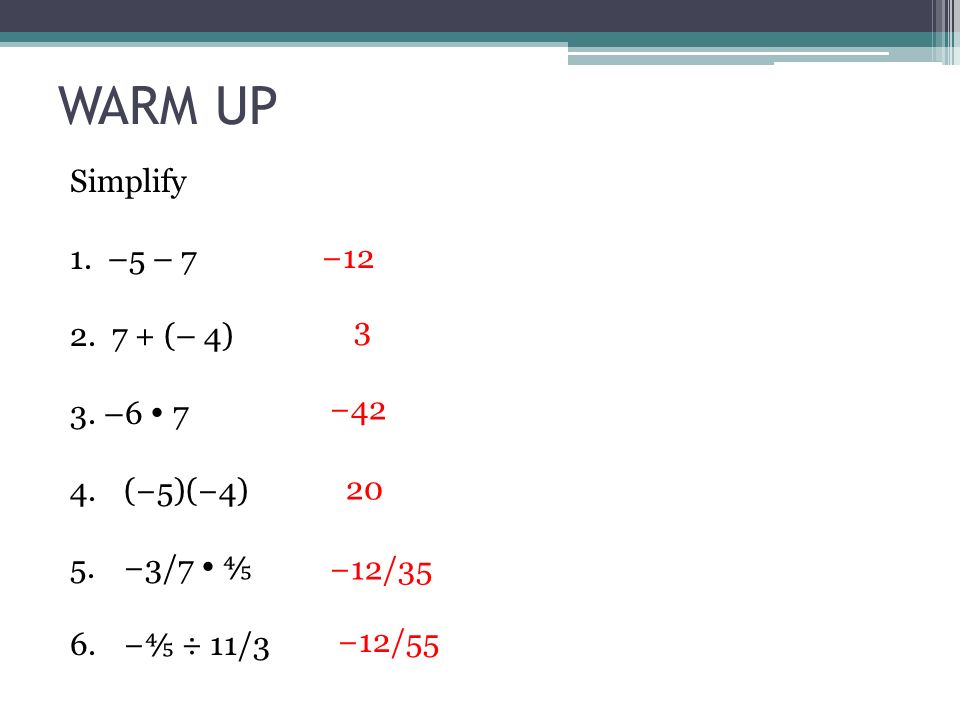
Warm Up Simplify 1 5 4 12 3 6 7 3 5 4 Ppt Video Online Download

Simplifying a square root isn't as hard as it looks To simplify a square root, you just have to factor the number and pull the roots of any perfect squares you find out of the radical sign Once you've memorized a few common perfectSimplify √32√2 Login Study Materials NCERT Solutions NCERT Solutions For Class 12 NCERT Solutions For Class 12 Physics; Surds are numbers that are placed in square roots and when expressed as a fraction, the values of their decimal form continue till infinity From the given information, we are to simplify the given surds to the smallest possible value ie = (2√53√7)^2 = (2√53√7) (2√53√7) Using the multiplication rule, we have = 4√25 6√

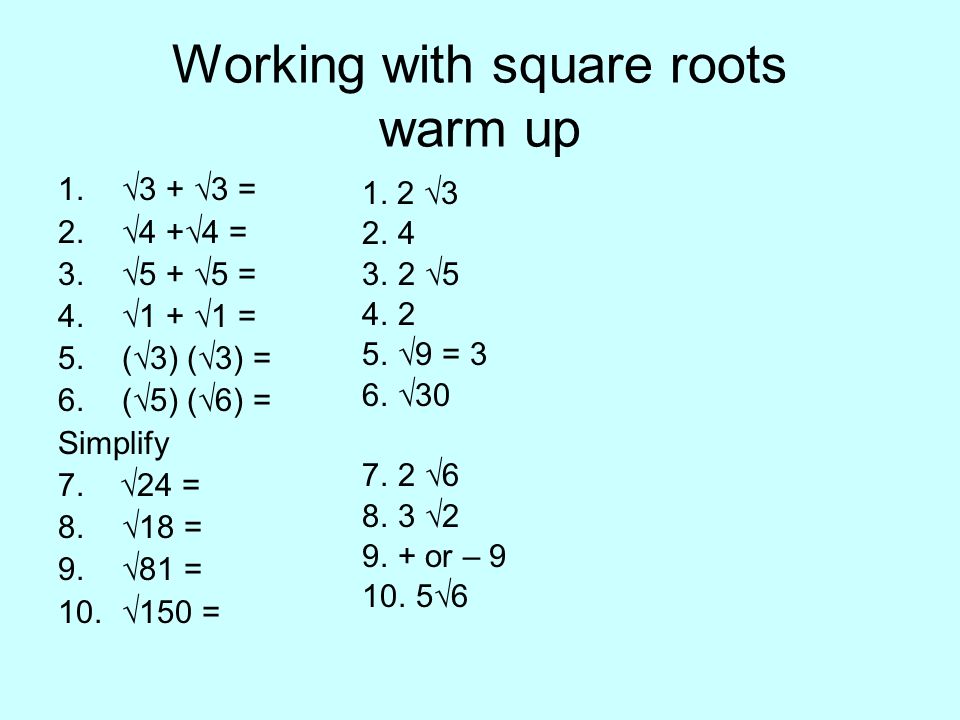
Working With Square Roots Warm Up 1 3 3 2 4 4 3 5 5 4 1 1 5 3 3 6 5 6 Simplify 7 24 8 18 9 81 10 Ppt Download

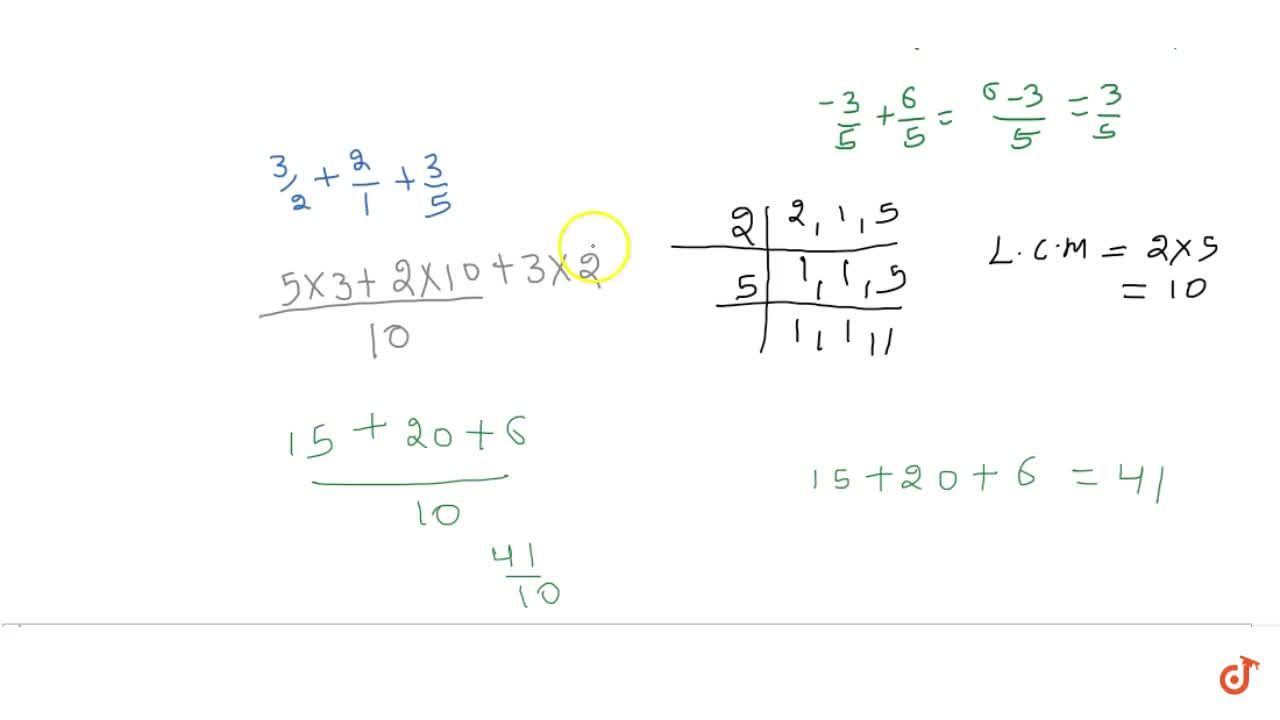
Simplify 3 8 7 2 3 5 9 8 3 2 6 5

A mixed number is an addition of its whole and fractional parts To write 6 6 as a fraction with a common denominator, multiply by 3 3 3 3 Combine 6 6 and 3 3 3 3 Combine the numerators over the common denominator Simplify the numerator Tap for more steps Multiply 6 6 by 3 3 Add 18 18 and 2 2 The result can be shown in multiple formsNCERT Solutions For Class 12 Biology;Simplify (2 √3)/(√3) CISCE ICSE Class 9 Question Papers 10 Textbook Solutions Important Solutions 15 Question Bank Solutions Concept Notes & Videos 441 Syllabus Advertisement Remove

Rationalize The Denominator And Simplify 2 3 2

Simplify 3 5 5 2 4 5 3 2 With Video Teachoo Rationalising

Rationalize the denominator and simply3 63 2 − 6 24 3 2 36 EasyHere is the rule when a and b are not negative Example simplify √6 × √15 First we can combine the two numbers √6 × √15 = √(6 × 15) Then we factor themGiven expression is 3 63 2 − 6 24 3 2 36On rationalizing each term and using the property (a−b)(ab)=a 2−b 2 for each denominator, we get(3) 2−(6) 23 2(3− 6) − (6) 2−(2) 24 3(6− 2) (2) 2−(3) 26(2− 3)= −33 6−3 12− 44 18− 6 −1 12− 18= 1212 12−12 6−12 1 6−12 1212 18= 12−9 6= 4−3 6Hence, 3 63

Simplify 3 6 4 6 Youtube

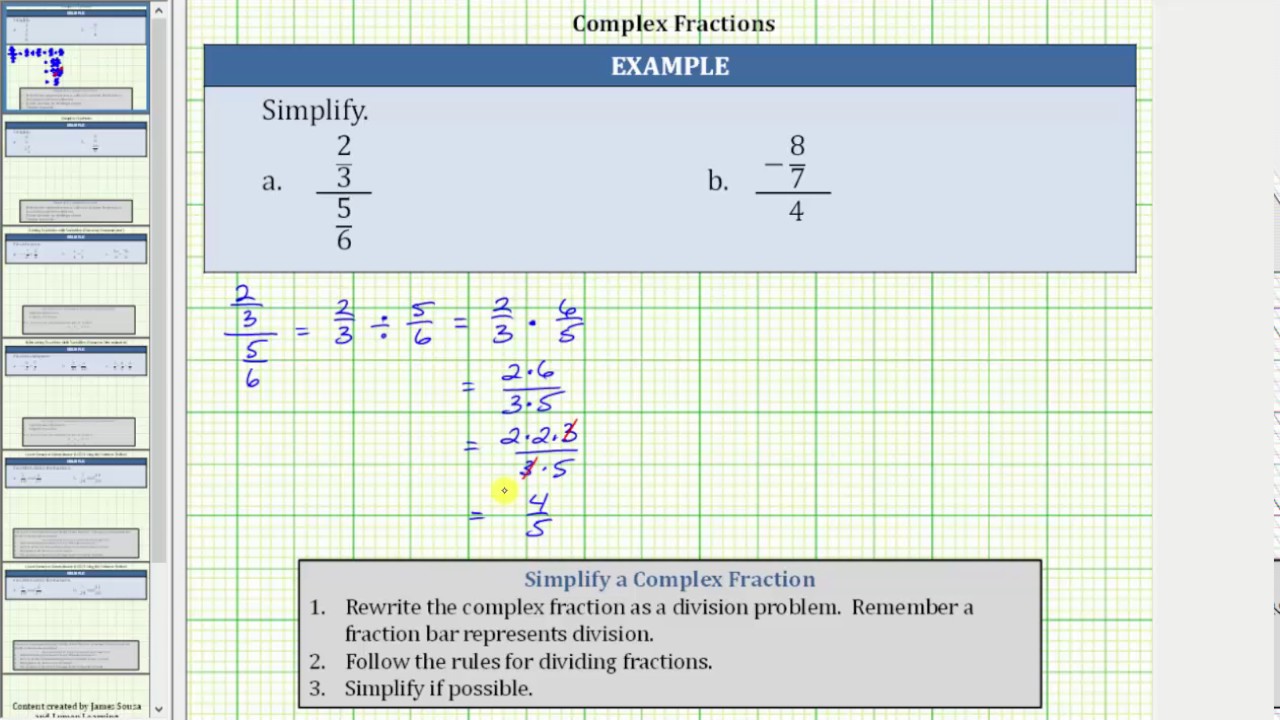
Simplify Complex Fractions 2 3 5 6 And 8 7 4 Youtube

Answer (3) 3√3 It is an example of adding two irrational numbers 2√3 √3 We can see that there are two terms that contain two √3, one with coefficient 2 and the other with coefficient 1, and there would be 2 1 = 3 On taking out √3 as common0 votes 1 answerIf a and ß are the zeroes of the polynomial t 4t 3, find the value of Alpha^4 beta^3 alpha^3 beta^4 8 If a and 3 are the zeroes of the quadratic polynomial x² (p

6 Simplify 3 2 6 3 2 3 6 2 4 3 6 2 Brainly In

Add 3 2 7 3 And 2 5 3 Rational Numbers Video Teachoo

Login Create Account Class9 » Maths Number Systems SIMPLIFY 2 √6 / √2 √3 6 √2 / Rationalising Rationalising denominator of irrational number Add (3√27√3) and (√2−5√3) Divide 5√11 by 3√33 Multiply 2√15 by 7√5 Simplify (√5√7)^2 Simplify (√4−√13) (√4√13) Simplify (9−√3) (9√3) Simplify (3√5−5√2) (4√53√2) Rationalise the denominator of 8/√7 Rationalise the denominator of 1NCERT Solutions For Class 12 Chemistry;

Simplify 2 30 6 3 140 28 55 99

How To Find 1 2 1 4 1 8 Video Lesson Transcript Study Com

Simplify √ 3 2 SIMPLIFY 2√6 / √2√3 6√2 / √6√3 8√3 / √6√2 Maths Number Systems NCERT Solutions;Simplify 3√2/√3 √6 4√3/√6√2 √6/√2 √3 asked Apr 19 in Number System by abcdefg4929 (15 points) class9;

Simplify I 3 Sqrt 11 3 Sqrt 11 Ii 3 Sqrt 5 3 Sqrt 3 Sqrt 5 Iii Youtube

Simplifying Square Roots Of Fractions Algebra Video Khan Academy

 We can simply solve this mathematical problem by using the following mathematical process (our goal is to simplify the given mathematical expression) Here, we will be using very basic methods (eg taking common) to simplify the given mathematical expression So, = 2√3 √3 = √3 (2 1) Taking √3 common from both the addends = √3 × 3 = 3√36 Simplify (3√3)(2√2)² Get the answers you need, now!Click here👆to get an answer to your question ️ Simplify 2√(3)3 √(3)6

B Simplify By Rationalizing The Denominators 1 Gauthmath

Simplify Each Of The Following Expressions I 3 Sqrt 3 2 Sqrt 2 Ii 3 Sqrt 3 3 Sqr Youtube

Use the Binomial Theorem Simplify each term Tap for more steps Apply the product rule to 2 x 2 x Raise 2 2 to the power of 3 3 Apply the product rule to 2 x 2 x Raise 2 2 to the power of 2 2 Multiply 4 4 by 3 3 Multiply − 3 3 by 12 12Solution We know that rationalization factor for 3 2 2 3 and 3 2 are 3 2 2 3 and 3 2 respectively We will multiply numerator and denominator of the given expression 3 2 2 3 3 2 2 3 and 12 3 2 by 3 2 2 3 and 3 2 respectively, to getNCERT Solutions Class 12 Accountancy;

Rd Sharma Solutions For Class 7 Maths Chapter 6 Exponents Download Free Pdf

Simplify Radical Expressions

 Simplify the radical expression √180x^2 90x** 6x√5 5x√6 6√5x^2 3 simplify he radical expression √250h^4 k^5 hk√125 5√10h^4 k^5 5h^2 k^2√10k** Algebra Simplify the radical expression √72x^2 A 36√x^2 B 6x √2 ***** C x√36 D 6√2x^2 algebra 1 simplify the radical expression √50 a 5√2 b2√5 c5√10 d5 2Solution 2√36/√33√27 = 2√36√3/(√3*√3)3*3√3 = 2√3–2/√39√3 = 9√3 AnswerNCERT Exemplar Class 9 Maths Exercise 13 Problem 9(vi) Simplify the following (√3 √2)² Summary Irrational numbers are the set of real numbers that cannot be expressed in the form of a fraction, p/q where p and q are integers

What Is The Simplified Form Of 12 6 3 The Square Root In Front Of The 12 Is Over The Whole Expression Including 6 3 Quora

Answer Page 1

Example √12 is simpler as 2√3 Get your calculator and check if you want they are both the same value!Simplify the Following √ 6 √ 2 √ 3 3 √ 2 √ 6 √ 3 − 4 √ 3 √ 6 √ 2 CISCE ICSE Class 9 Question Papers 10 Textbook Solutions Important Solutions 15 Question Bank Solutions Concept Notes & Videos 436 Syllabus AdvertisementHow do you simplify 2√36/√33√27?

Simplify 2 3 6 3 2 4 Brainly In

Simplify Sqrt 2 Sqrt 6 Sqrt 2 Sqrt 3 Sqrt 6 Sqrt 2

 Simplify fully 2√3 x 3√8 Get the answers you need, now!0 votes 1 answer Simplify 1/√3√2 1/√3√2 asked Mar 24 in Number System by PraveenMeena (452k points) real numbers; Equivalent Algebraic Expressions The game shown at the right consists of eight pairs of coloured squares called dominos Rules 1 Write a polynomial in each square marked P and a rational function in each square marked R 2 The expressions you write must

61 28 3 A B C How To Find A B And C The First Square Root Is For All Of 61 28 3 Quora

Simplify 2 45 3 2 5 And Clear Whether It Is Rational Or Irrational Number Sarthaks Econnect Largest Online Education Community

Simplify 6 2 3 2 Brainly In

Simplify By Rationalising The Denominator 2 Root 6 5 3 Root 5 2 Root 6 Youtube

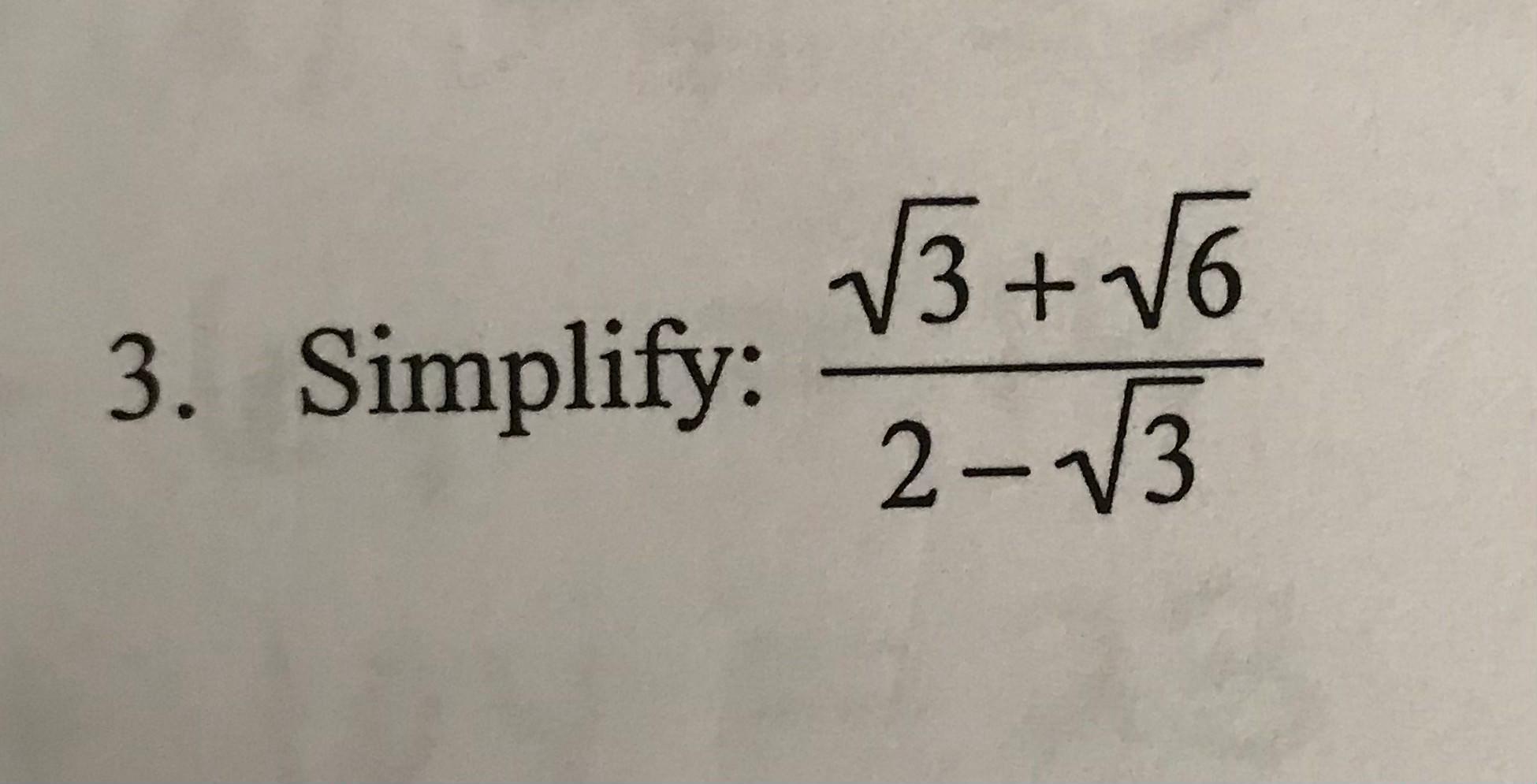
Solved 3 Simplify 3 6 2 13 Chegg Com

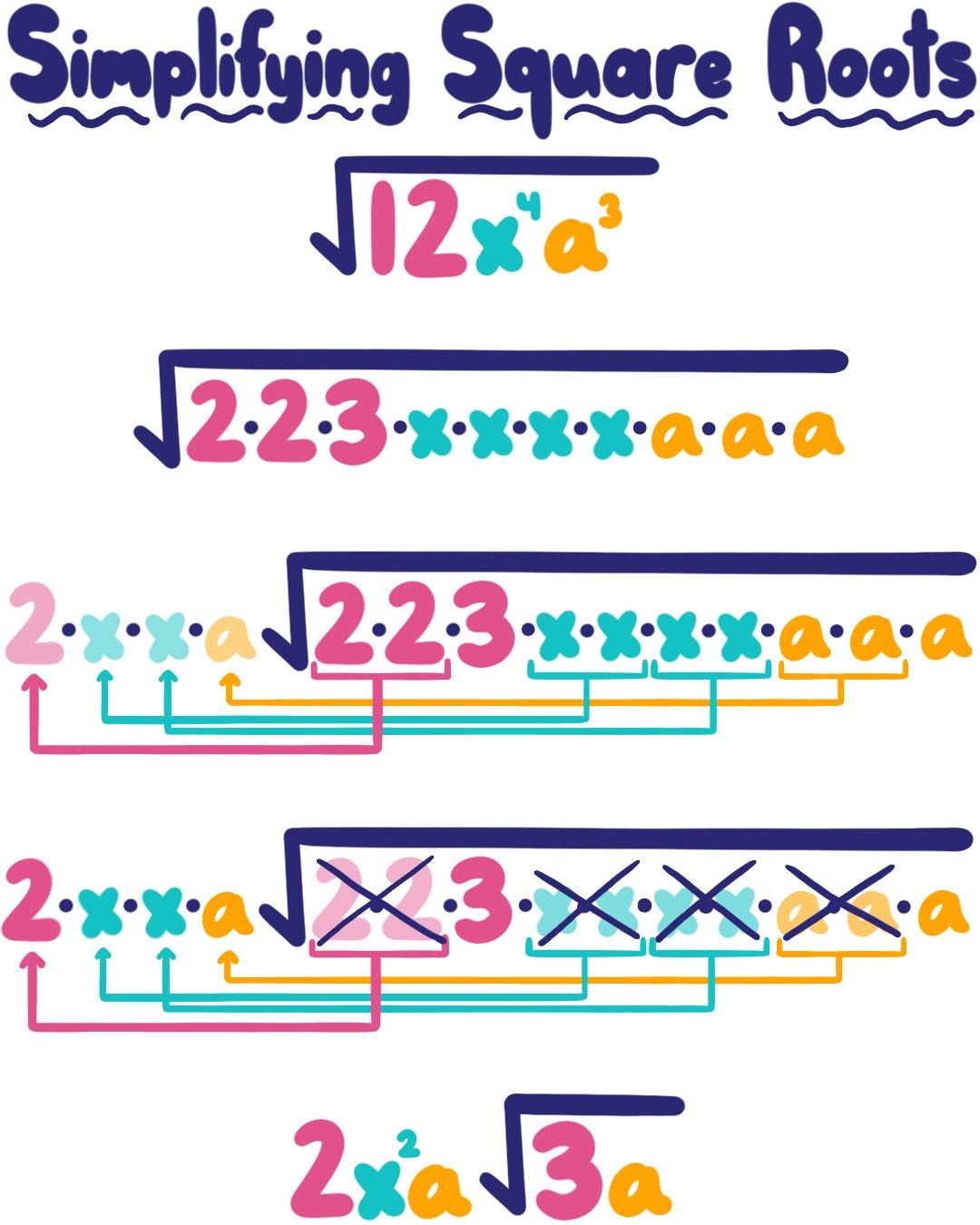
Simplifying Square Roots Examples Practice Expii

How To Simplify Math Frac Sqrt 3 6 Sqrt 3 10 Sqrt 4 2 Sqrt 3 Math Quora

Simplify 15 Simplify 7 310 3 2 56 5 3 215 3 2 Maths Number Systems Meritnation Com

Rationalize 1 7 3 2 Maths Questions

Solved Find The Function Value X 3 1 F X F 2 1 X Chegg Com

7 3 10 3 2 5 6 5 3 2 15 3 2 Maths Number Systems Meritnation Com

Can We Add 2 And 3 If So Then What Will Be The Answer Quora

Real Numbers And Radicals

2 Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 Youtube

Simplifying Square Root Expressions Video Khan Academy

Simplify 3 2 6 3 2 3 6 2 4 3 6 2 Brainly In

Simplify 2 3 6 4 3 6 2 6 2 3 Brainly In

3 8 By 3 2

Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2

Simplify Each Of The Following Expressions 3 3 2 2 3 3 3 3 5 2 5 2 5 2 Youtube

Section 6 2 Day 1 Apply Properties Of Rational Exponents Ppt Download

What Is The Solution Of 12 6 3 8 2 8 6 Quora

Simplifying Square Root Expressions Numbers With A Root

Simplify 3 5 5 2 4 5 3 2 With Video Teachoo Rationalising

Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2

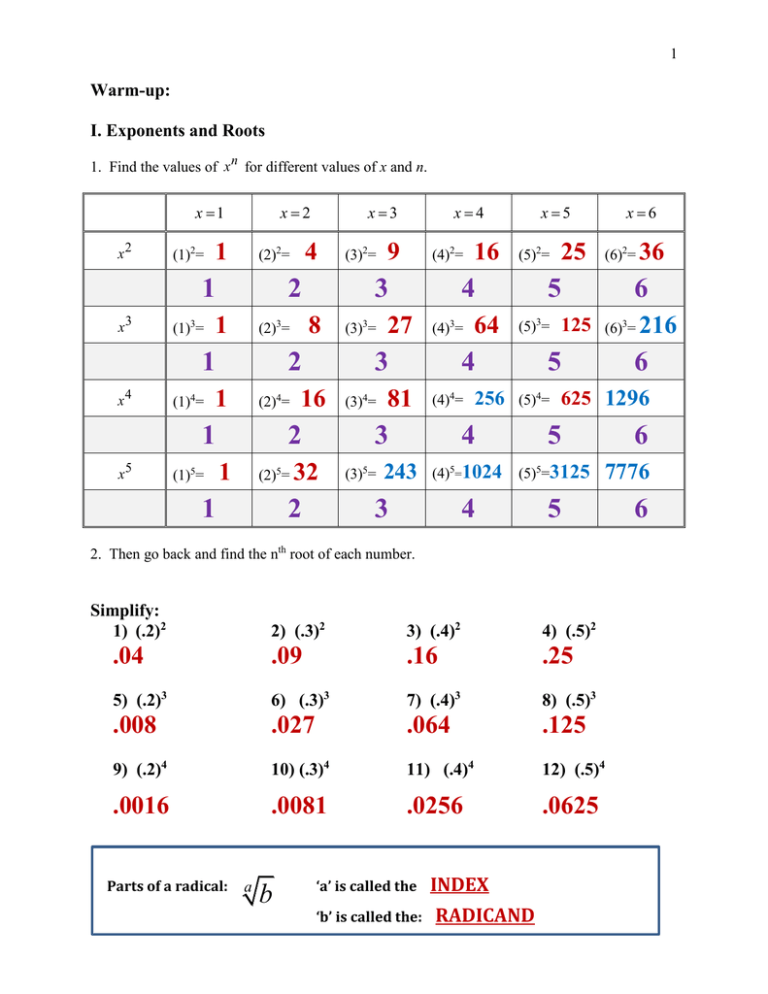
Exercise Find The Prime Factorization Of 700 22 52 7 Ppt Download

Ex 1 6 3 I Simplify 2 2 3 2 1 5 Class 9 With Video

3 Ways To Multiply Radicals Wikihow

Example 12 Simplify I 12 4 9 3 4 6 3 8 2 27 Class 7

Simplify And Rationalise The Denominator Of 6 4 2 6 4 2

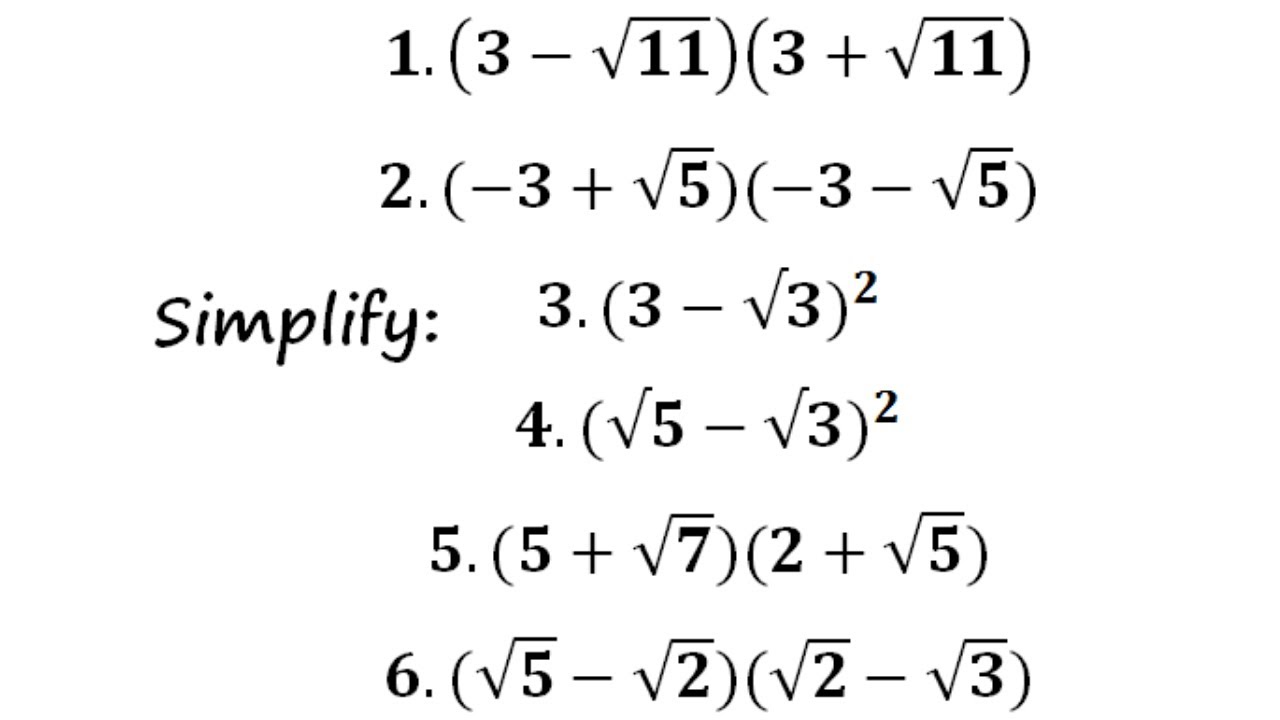
Simplify 1 3 11 3 11 2 3 5 3 5 3 3 3 24 5 3 25 5 7 2 5 6 5 2 2 3 Youtube

Simplifying Square Roots Algebra Video Khan Academy

Simplify 2 Root 3 By 3 Minus Root 3 By 6 Brainly In

Rd Sharma Solutions For Class 7 Maths Chapter 6 Exponents Download Free Pdf

Document

What Is The Answer To 2 3 Quora

What S The Correct Way To Solve These 3 7 6 4 5 2 And 5 3 6 9 5 2 Quora

Simplify I 4 7 147 3 8 192 1 5 75 Ii 5 3 2 27 1 3 Iii 216 5 6 294 3 6 Sarthaks Econnect Largest Online Education Community

Simplify 3 5 3 2 5 3 Youtube

1

Simplifying Square Root Expressions No Variables Video Khan Academy

Simplify 3 3 2 3 2 6 3 Brainly In

Multiply 2 6 By 3 3

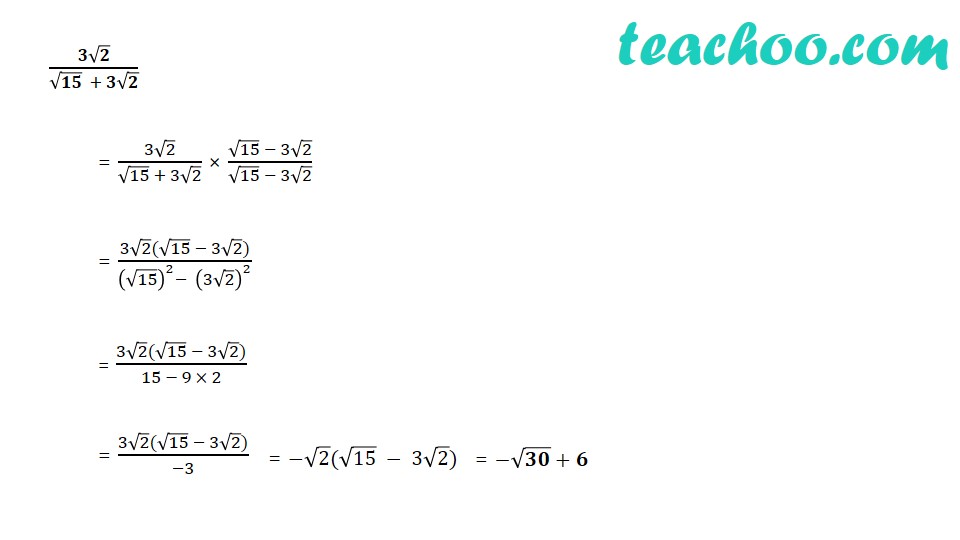
Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 V

Simplify 6 2 3 3 6 3 2 4 3 6 2 Brainly In

Simplify 6 2 3 6 6 3 2 Brainly In

Simplify 2 6 2 3 6 2 6 3 8 3 6 3 Brainly In

How To Simplify 6 3 2 Quora

6 Sqrt 27 3 Sqrt 3 1 2sqrt 3 When Simplified Is

Simplify Each Of The Following Expressions I 3 3 2 2 Ii 3 3 3 3 Iii 5 2 2 Iv 5 2 5 2

What Is 6 12 3 6

Simplify The Following 7 18 9 8 12 32 3 50

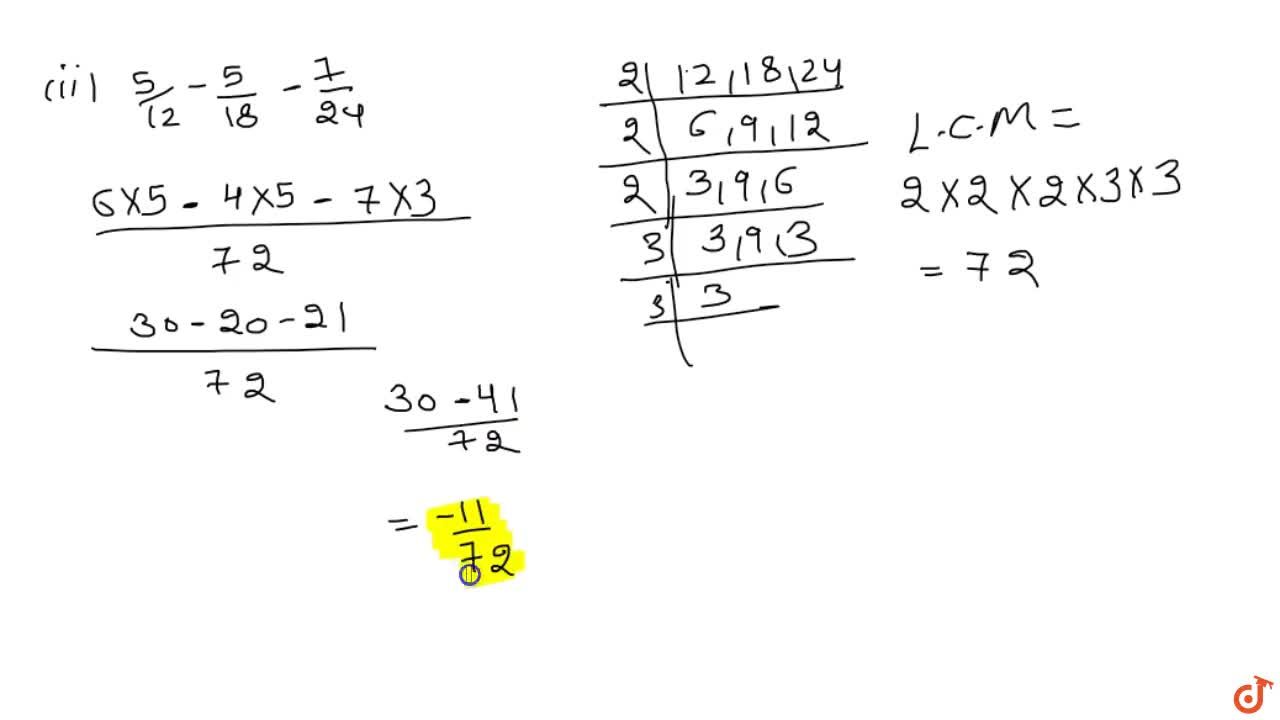
Simplify 2 3 5 9 7 6 Ii 5 12 5 18 7 24

Solved Simplify The Following Multiplication Problems No Chegg Com

After Rationalizing The Denominator Of 2 3 5 2 2 3 3 And Simplifying We Get A 3 15 B 10 4 6 19 Then The Value Of A B Is

How Would You Simplify Math Sqrt 2 Sqrt 2 Sqrt 3 Sqrt 6 3 Sqrt 2 Sqrt 3 Math Quora

Simplify I 4 7 147 3 8 192 1 5 75 Ii 5 3 2 27 1 3 Iii 216 5 6 294 3 6 Sarthaks Econnect Largest Online Education Community

What S The Correct Way To Solve These 3 7 6 4 5 2 And 5 3 6 9 5 2 Quora

Solved Question 31 Simplify 3 1 3 31 4 10 31 4 5 31 Chegg Com

Simplify 2 3 3 3 6 Youtube

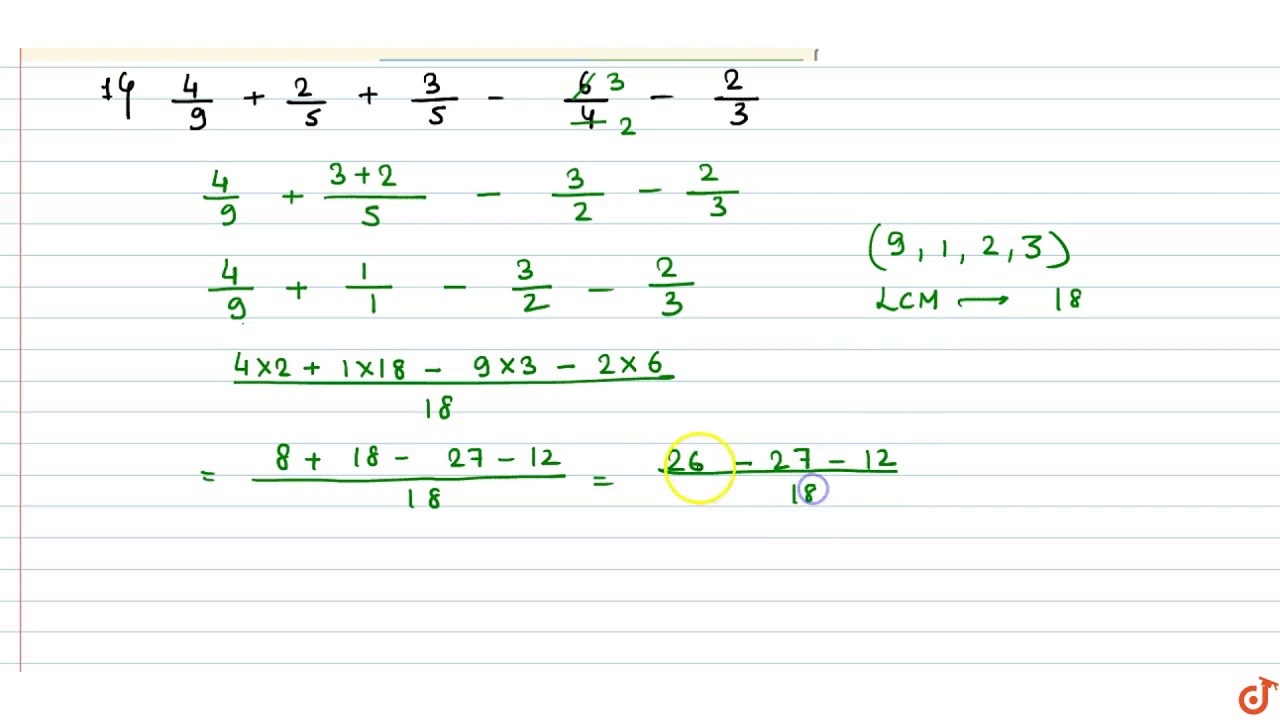
Simplify The Expressions 1 4 9 2 5 3 5 6 4 2 3 2 11 2 8 3 4 2 5 Youtube

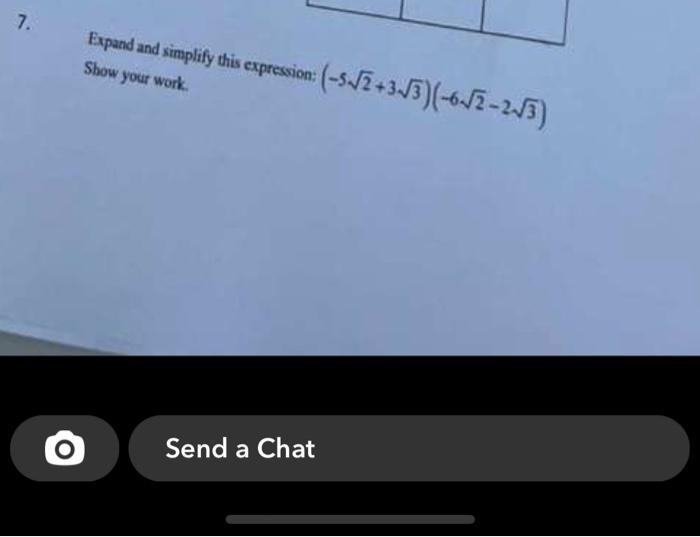
7 Expand And Simplify This Expression Chegg Com

Simplify Simplify Radical Rational Expression With Step By Step Math Problem Solver

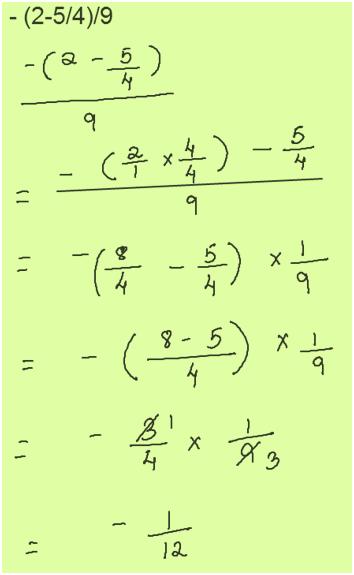
Free Math Answers Answers Within 24 Hours Step By Step Explanations

Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2

Simplify 2 3 3 3 6 Brainly In

Q 28 A Simplify Frac 6 2 Times 5 3 Times 4 3 4 2 Times 5 4 Times 6 3 B By What Number Should 5 1 Multiplied To Get25 1 29 A Simplify 7x 2 3x 9 3 And Find Its Value For X 6 Snapsolve

Simplify 2 3 A 3 5a 4 Exponents And Powers Class 7

Simplify 2 Root 6 By Root 2 Root 3 Rs Aggarwal Class 9 Exercise 1f Question 07 Iv Youtube

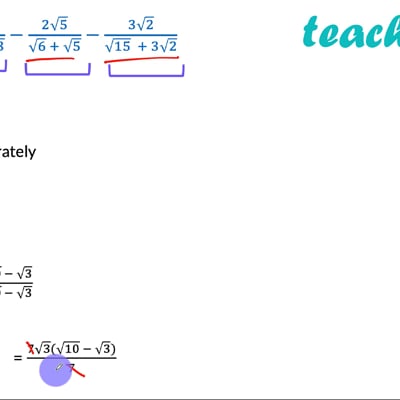
Simplify 7 3 10 3 2 5 6 5 3 2 15 3 2 V

Incoming Term: simplify 2 root 3/3-root 3/6, simplify 3^2 x 3^-5 x 3^6, simplify 2 root 3 by 3 minus root 3 by 6, simplify 3^-6x(3^4/3^0)^2,